Well you must like the look of green and yellow cable !Yes, anything which had the potential to accidentally live.
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Lack of supplementary bonding - what’s the danger ?
- Thread starter equitum
- Start date
Sponsored Links
- Joined
- 27 Jan 2008
- Messages
- 25,080
- Reaction score
- 2,915
- Location
- Llanfair Caereinion, Nr Welshpool
- Country

I have worked on an IT system, a tunnel boring machine (Hong Kong), shocks all the time even with in the main an all metal machine, and have also worked on Farms on the Falklands with lack of earths and bonding, I think it was thought since only a 1.5 kVA generator not required, and yes got shocks. So there is good reason to earth and bond things, but both the cases above no RCD protection.
The reliance of RCD protection is also a problem, even type A RCD's only rated for 6 mA DC, so in real terms rather easy for the RCD not to do what is required, to my mind the RCBO is the answer as then limited what will be unprotected if there is DC around.
However we need to ask ourselves the question.
Are the more than one equipotential zones? if so can these be bonded together without under fault conditions excessive currents flowing? If excessive currents will flow, can the zones have enough distance between them so we have under fault conditions a safe voltage gradient?
2 meters seems to be considered as the safe distance, seems a bit close to me, but this does include any item which can bridge the gap, so for example if a caravan is 2 meters, and is likely to be plugged into the supply one meter from the caravan, then it would need 5 meters from the TT caravan supply to the TN-C-S building supply to ensure there is 2 meters between the earthing systems.
I can see that an un-bonded central heating copper pipe can transmit a fault between rooms, but that is not really the case with metal window frames, and had the main stop tap not been earthed with the Emma Shaw case she may have still been alive, and also if the metal in the walls had be bonded she would still be alive, or if RCD's had been fitted. However poor inspection and testing was blamed.
We should use a low ohm meter using at least 200 mA to test bonding, but when is this really done? Does the plumber actually test if the joint plastered with boss white is still able to bond?
The reliance of RCD protection is also a problem, even type A RCD's only rated for 6 mA DC, so in real terms rather easy for the RCD not to do what is required, to my mind the RCBO is the answer as then limited what will be unprotected if there is DC around.
However we need to ask ourselves the question.
Are the more than one equipotential zones? if so can these be bonded together without under fault conditions excessive currents flowing? If excessive currents will flow, can the zones have enough distance between them so we have under fault conditions a safe voltage gradient?
2 meters seems to be considered as the safe distance, seems a bit close to me, but this does include any item which can bridge the gap, so for example if a caravan is 2 meters, and is likely to be plugged into the supply one meter from the caravan, then it would need 5 meters from the TT caravan supply to the TN-C-S building supply to ensure there is 2 meters between the earthing systems.
I can see that an un-bonded central heating copper pipe can transmit a fault between rooms, but that is not really the case with metal window frames, and had the main stop tap not been earthed with the Emma Shaw case she may have still been alive, and also if the metal in the walls had be bonded she would still be alive, or if RCD's had been fitted. However poor inspection and testing was blamed.
We should use a low ohm meter using at least 200 mA to test bonding, but when is this really done? Does the plumber actually test if the joint plastered with boss white is still able to bond?
I'll see if I can find a diagram.Because my question is, I’d like to know a scenario where touch voltage is going to exceed let’s say 50 V main bonding is in place and all circuits in the location have a CPC
Indeed - as I wrote.I was about to make the point that if the metal waste pipe and trap were indeed continuous into the ground they would be /should be main bonded .
ditto.So we are down to shock protection during a LE fault duration of typical 0.4s for final circuits.
I would imagine the traditional thinking is that any electric shock is likely to be more serious (higher current), hence more likely to prove fatal, when people are 'naked and wet'. With west skin, body resistance can be 1kΩ or lower, hence at 230V can result in a shock current of 230 mA or more, which might be fatal,even if a ("30mA") RCD limited to duration to a couple of dozen milliseconds.Thinking aloud, is there anything more dangerous during this period in a zone than in say a bedroom because we are talking 240V touch voltage here - maybe more exposed pipe work - but a radiator would have the same effect I’m sure in another room .
Having said that, scenarios such as flameport suggested are not restricted to bathrooms. Some of what I recently removed was a lead waste pipe and trap in a bedroom basin - a situation in which water might also be wet.
Without supplementary bonding, even though all CPCs are joined at the MET, inb the event of an L-E fault the potential of one of them (hence any associated exposed-c-ps) will rise to very much higher than MET potential, whereas the potential of COPCs/exposed-c-ps of other circuits or main-bonded extraneous CPs will remain at MET potential - hence a potentially dangerous potential difference.In any case what is the supp bonding achieving here that isn’t already achieved by all of the CPC’s being combined at the CU and all of the extraneous services being connected be main bonding connections?
Sponsored Links
As I've just written ....I’d still be interested to understand the object of it and the scenarios they were intending to mitigate because I can’t get my head around the usual “to prevent PD between extraneous conductive parts” whilst the zone occupant is performing some odd pose whilst wet and unrobed. I’ve always wondered how large this PD could possibly be if main bonding in place, and in all likelihood even if it isn’t ?
If there is an L-CPC fault in the room, then the potential of that CPC in the room (hence also the potential of associated exposed-c-ps) will rise to a relatively high voltage (relative to MET), determined only by the ratios of the CSAs of L conductor and CPC in the circuit. For example, with a 230V supply and 2.5/1.5mm² cable, in the case of an L-CPC fault in the room, it';s potential will rise to about 144V above MET potential.
Since the exposed-c-ps of things on any other circuits, or of main-bonded extraneous-c-ps (or things in electrical continuity with them) will remain at MET voltage, there will then be a high potential difference (about 144V) between them and exposed-c-ps of the circuit with a faukt.
As I've said, nothing other than local supplementary bonding can prevent that.
Couldn't find any suitable diagram so - is this any help?
What will be the voltage between points A and B with and without the supplementary bonding.
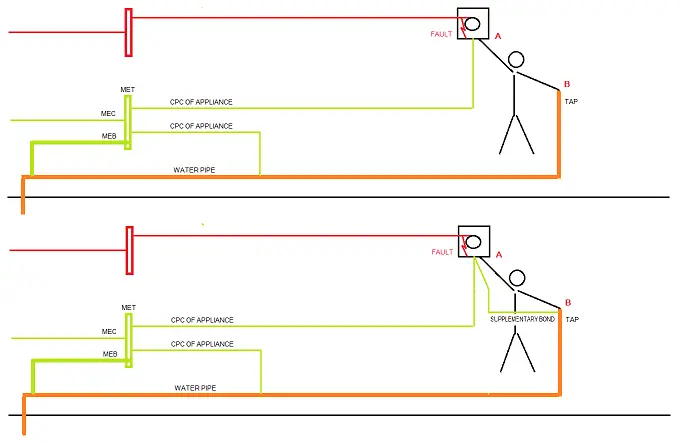
What will be the voltage between points A and B with and without the supplementary bonding.
As I've recently written ...Couldn't find any suitable diagram so - is this any help? .... What will be the voltage between points A and B with and without the supplementary bonding.
Without SB, the pd between A & B would depend upon the circuit's cable (ratio of L & CPC CSAs) but, for example, with 2.5mm² T+E, it would be about 144 V.
With SB, the PD between A & B would be fairly close to zero - small deviations from zero being due to the 'voltage drop' in the (presumably short, and of reasonable CSA) SB conductor due to it carrying a portion of the fault current.
What’s your calculation to achieve 144V ? I assume 240/1.667 ?
In the top picture, from another forum poster: If the resistance between point A and B is 0.25ohm then with a fault current of 200 A causing instantaneous trip of the OPCD then the voltage would be 50V ?
In the top picture, from another forum poster: If the resistance between point A and B is 0.25ohm then with a fault current of 200 A causing instantaneous trip of the OPCD then the voltage would be 50V ?
Last edited:
Yes but you are ignoring the pipe and its associated CPC.Depends on the magnitude of the fault current and the resistance of the cpc between A and the MET and R1 ? (Assuming negligible resistance of the supp bond)
If you assume they are negligible as well then the person is just touching two points close together on the same conductor - like the birds.
Yes, but I was asking Equitum for him to say; not because I didn't know.As I've recently written ...
Without SB, the pd between A & B would depend upon the circuit's cable (ratio of L & CPC CSAs) but, for example, with 2.5mm² T+E, it would be about 144 V.
With SB, the PD between A & B would be fairly close to zero - small deviations from zero being due to the 'voltage drop' in the (presumably short, and of reasonable CSA) SB conductor due to it carrying a portion of the fault current.
Ratio of CSA's of CPC and L conductor (of 2.5mm² T+E) is 1.5 : 2.5, hence ratio of R2 to R1 is 2:5 : 1.5.What’s your calculation to achieve 144V ?
Voltage across R2 = 230 x [ R2 / (R1+R2) ]
= 230 x [2.5 /4.0)
= 143.75
Oh yes, sure you were my whole original post was around in a dwelling, the differences would be negligible due to relatively short lengths of cpc and parallel paths with the ECPs and main bonding for supplementary bonding to make a differenceYes, but I was asking Equitum for him to say; not because I didn't know.
Fair enough - that wasn't clear (at least, not to me!)Yes, but I was asking Equitum for him to say; not because I didn't know.
lolFair enough - that wasn't clear (at least, not to me!)
DIYnot Local
Staff member
If you need to find a tradesperson to get your job done, please try our local search below, or if you are doing it yourself you can find suppliers local to you.
Select the supplier or trade you require, enter your location to begin your search.
Please select a service and enter a location to continue...
Are you a trade or supplier? You can create your listing free at DIYnot Local
Sponsored Links
Similar threads
- Replies
- 19
- Views
- 5K
- Replies
- 10
- Views
- 5K
